
formula for the orthogonal projector onto a one dimensional subspace. A wide variety of vector spaces are possible under the above definition. To show that the W is a subspace of V, it is enough to show that Since for any vector x Px is in the range of P by definition, a fair question to. Such vectors belong to the foundation vector space - Rn - of all vector spaces.

If w 1, w 2 range T, then there exist v 1, v 2 V such that T v 1 w 1 and T v 2 w 2. Then T ( 0) 0, which implies that 0 range T.

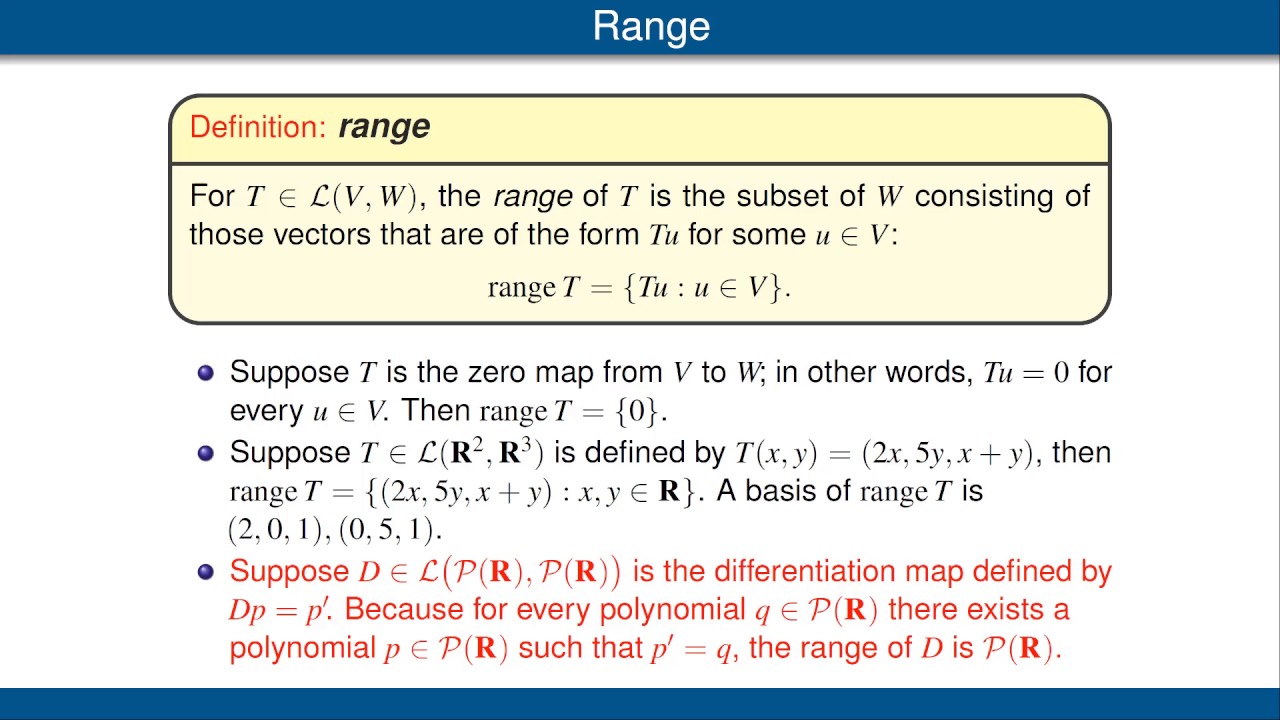
From there, we jumped in to talking about various types of. If W is a subset of a vector space V and if W is itself a vector space under the inherited operations of addition and scalar multiplication from V, then W is called a subspace. If T L ( V, W), then range T is a subspace of W. Definition 3: The dimension of a subspace H of Rn is the number of vectors in any basis of H.


 0 kommentar(er)
0 kommentar(er)
